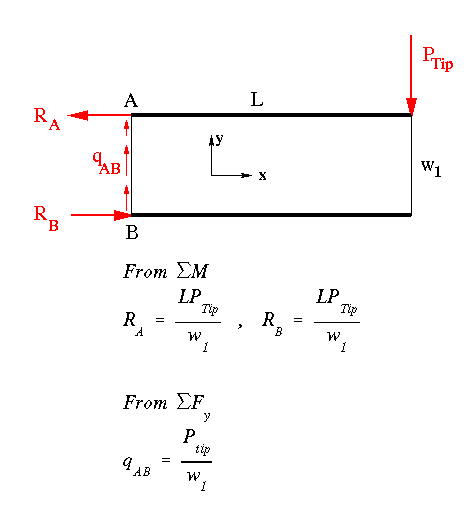
In tapered web-stringer sections, the stringers relieve some of the shear load carried by the webs. This can be shown by the following example.
Below is a planview of a flat, rectangular stiffened web. By simple summation of moments and forces, the axial forces in the stringers and shear flow in the web can be determined.
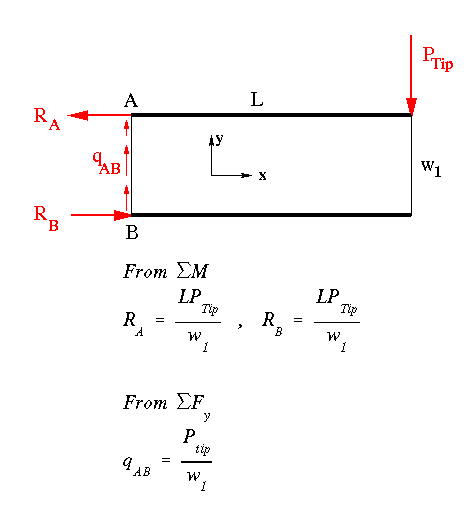
Now, for a tapered stiffened web of the same length we have
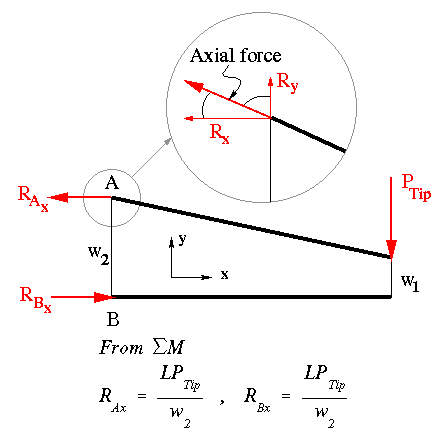
We observe that while stringer B has no slope in x-y plane, stringer A does. As a result, the axial force in stringer B is the same as its x component, while the axial force in stringer A is the summation of its x and y components shown above. As shown below, the shear force in the web at the left end is no longer equal to Ptip as in the untapered section, but rather is less - with the difference picked up by stringer A.
In tapered box beams we must pay close attention to the slope of each stringer to determine its axial force. Also in tapered webs the shear flow is no longer the same along two adjacent edges, as is the case with untapered webs.
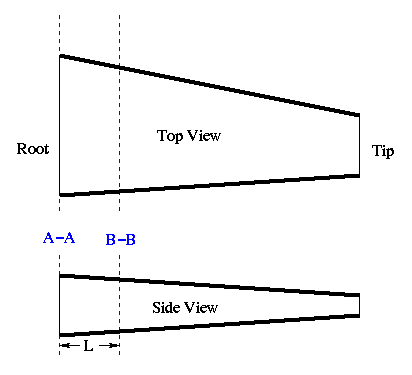
With respect to the use of Delta P method for calculating the shear flow distribution at a desired section, we realize that the accuracy of the method is decreased if the two adjacent sections along the beam (i.e., sections A-A and B-B shown in the figure below) are too far apart. This is especially true when we have a beam with nonuniform taper (one with the stringers and webs changing dimensions at a different rate from root to tip).
To Section
IV.5
To Index page of
Transverse Shear Loading of Closed Sections